Overview
This is a demonstration of the basic principles underlying the behavior of rotating bodies. A cylinder “race” is used to show that the closer the mass of an object is concentrated to an axis of rotation, the faster it will spin because it has a lower moment of inertia, which is a measure of a body’s resistance to rotation. The video below provides a brief synopsis of the demonstration.
Principle
The mass moment of inertia (pg. 1296 of the McGraw-Hill Vector Mechanics for Engineers—Dynamics text) is a rigid body’s resistance to rotation and is a measure of the distribution of mass of a rigid body relative to a given axis of rotation. In its most general form, the mass moment of inertia is given by:
where:
“I” is the mass moment of inertia
“dm” is a differential element of mass of the rigid body
“r” is the perpendicular distance from the axis of rotation to a differential element of mass
“B” represents the rigid body
For a solid cylinder and a hollow cylinder, the equations for the mass moment of inertia about the axis of interest in our demonstration reduce to those in figure 1 (http://hyperphysics.phy-astr.gsu.edu). “M” represents the mass of the rigid body and “R” represents the radius of the solid cylinder, and “a” and “b” represent the inner and outer radii of the hollow cylinder.
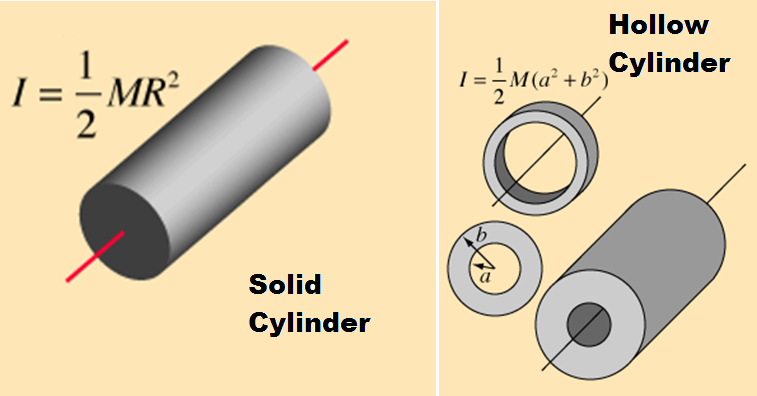
Figure 1: Mass Moment of Inertia
What You Need
These materials can be easily manufactured. Additionally they (or similar materials) can be obtained from businesses that specialize in building teaching aids, such as Arbor Scientific.
| Item | Quantity | Description/Clarification |
|---|---|---|
| Inclined Plane | 1 | An inclined plane able to support both rolling cylinders simultaneously (Figure 2) |
| “Steel Wheel” | 1 | A steel cylinder of approximately 3” length and 1” diameter with a wooden core which weighs approximately the same as “Rolling Timber” (Figure 3) |
| “Rolling Timber” | 1 | A wooden cylinder of approximately 3” length and 1” diameter with a steel core which weighs approximately the same as “Steel Wheel” (Figure 3) |
| 12″ Ruler | 1 | Used to start the race |

Figure 2: Inclined Plane

Figure 3: Steel Wheel (left) and Rolling Timber (right)
How It’s Done
Before Class: Obtain materials. Measure and calculate the basic parameters (mass, diameters). Practice demonstration.
In Class: First establish the scenario by introducing and describing “the players” in your best race announcer’s voice. Then, without any analysis, ask the students to guess which “player” will win the competition. Ensure you record this on the board. Build up to the start of the race and stop just short of letting them go. Ask students to consider if weight might be a factor affecting the outcome, then provided the class with the weight of each player and see if their guesses change (record on the board). Again, build up to the start and stop short to discuss the concept of mass being a resistance to translation and moment of inertia as resistance to rotation. Have students help you calculate the moment of inertia for each of the players (figure 4) and take a final tally of the bets. Finally, let the race happen!
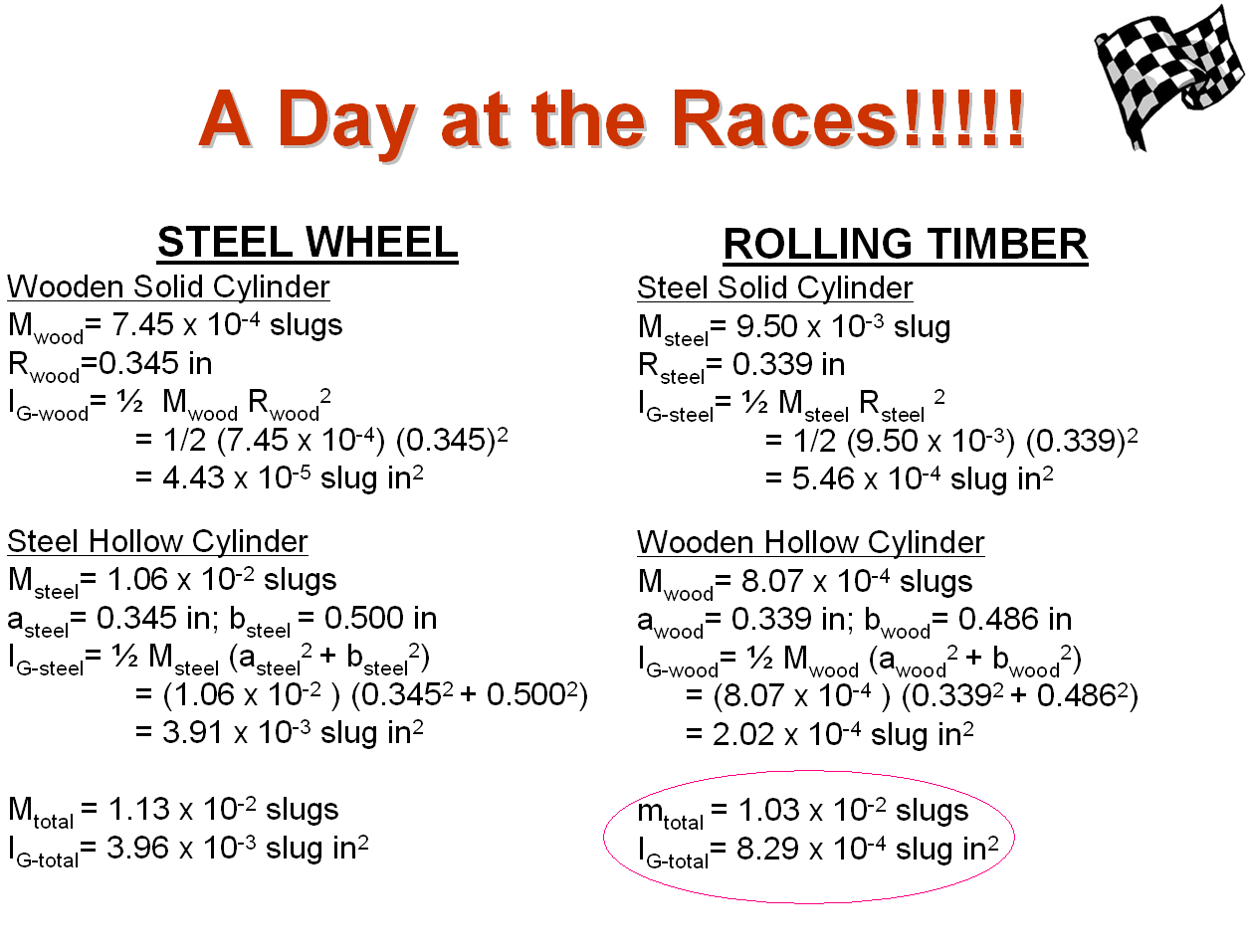
Figure 4: Calculation of Inertia
Observations: The student should observe that the outcome is a factor of the moment of inertia and not the weight (given the overall dimensions and the weight of the players are approximately the same.
That Little Extra
Hype up the event by dramatizing the race! Consider using racetrack videos and noises which can easily be found on the internet. Ask students to guess which will win once the scenario is set up and before the principles are discussed. Build suspense by working your way to the “gun shot” starting the race, then backing off the start to analyze another aspect. If you’re using Power Point, incrementally build your slides to build suspense!
Furthermore, cylinders of different materials and diameters might be considered (figure 5).

Figure 5

